Mathematics as a body of knowledge has expanded exponentially since the beginning of the 20th century. Historian of mathematics, E. T. Bell said that Henri Poincaré (1854–1912) was probably the last mathematician who understood all of the fields of mathematics during his time. Since then, mathematics, developed in academia and in industry has exploded in scope and depth. The accelerated rate of change that occurred in computer technology during the 1960’s and 70’s spiked in the 1980’s into an explosive growth in computing power. The computer even revolutionized the concept of “proof”–the cornerstone of mathematical certainty for over 2000 years. The Four-Color Conjecture that had eluded proof for over a century was finally proved by a computer in 1976 and proof by computer became an accepted process.
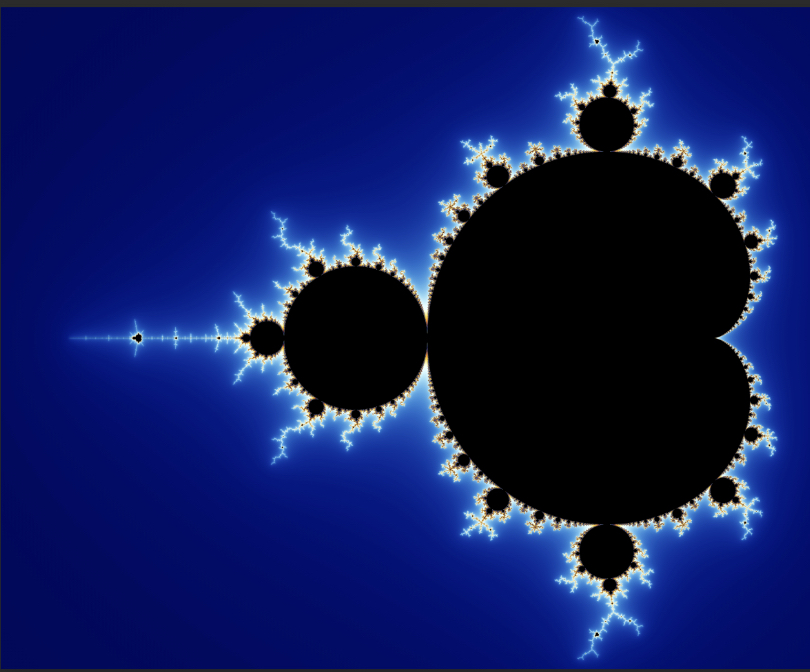
In 1979, maverick mathematician, Benoit Mandelbrot, while working at IBM’s Thomas J. Watson Research Center, took inspiration from an idea of Henri Poincaré, and wrote a computer program to explore the graph that results when the point 0 + 0i in the complex plane is mapped repeatedly under the transformation defined by z → z^2 + c where c is a complex number. This involves taking the point 0 + 0i in the complex plane, squaring it, adding c and then iterating this process, ad infinitum, to generate a sequence called the orbit of c. On March 1, 1980, Mandelbrot first glimpsed at the vague outline of what has become known as the Mandelbrot set, shown above. This exceedingly complex structure that emerged from that simple iteration ushered in what is now called the mathematics of chaos. When we “zoom in” on this figure, we discover that the boundary of the Mandelbrot set is infinitely “crinkled.” As the value of c changes imperceptibly, the orbit of c changes from a convergent to a divergent series. Mandelbrot and others showed that changing, by a minuscule amount, the initial conditions in a non-linear dynamic system can result in dramatic changes in the outcomes. Lorenz and others had studied weather patterns and realized that many systems in nature can best be described with computer models.
Today, we have a wide variety of new fields of mathematics including mathematical biology, algebraic geometry and string theory, topological data analysis and random matrix theory. This means that anyone interested in doing mathematical research must confine themself to a relatively narrow field and spend a significant amount of time in acquiring a strong mathematical background, before reaching the frontiers of that field. In this sense, mathematics has raised the entry-level bar for research.
However, if we are talking about school mathematics (K – 12) the level of mathematics presented is not as difficult as it was a century ago. The reason is simply a matter of demographics. In the 1930’s a much smaller percentage of the population completed high school, and those who did were usually the most capable students. Today, we enter almost the entire population of young people into high school, including those who have learning difficulties. This resulted in a “dumbing down” of the mathematics curriculum and the postponement of concepts like proof in Euclidean geometry to much later grades. To compare the grade 8 curricula of the past and present in algebra, look at the Jersey City High School Entrance Exam of 1885 shown below.
This doesn’t mean that people are less capable than in the past, it merely means that we’re educating a broader sector of our population and consequently, the pre-university levels of education have been reduced in difficulty to accommodate a broader range of learning abilities. This is not a problem as long as we ensure that the most capable learners are provided with a more rigorous program.
