There are eight teams in a junior hockey league and during hockey season, every team plays each of the other teams exactly once. How many games do the teams play during the season?
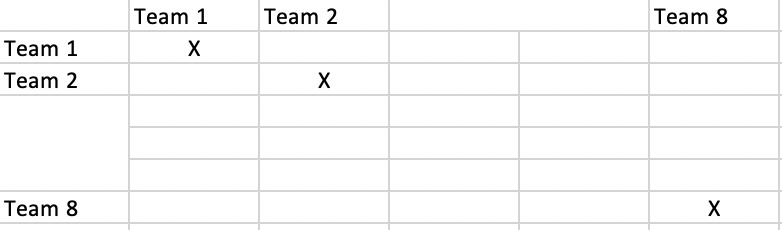
Answer: The grid below shows 8 rows of 8 columns. The cell in row 3 column 2 represents a game between team 2 and team 3. The grid shows 8 x 8 or 64 matches. However, a team does not play a game against itself so all eight diagonal cells are not true matches, leaving one 64 – 8 or 56 games. However, the grid matches each team twice with each of the other teams so we must remove half of the 56 games in half, leaving only 28 games. For those with a knowledge of combinators, the answer is directly computable as 8C2.