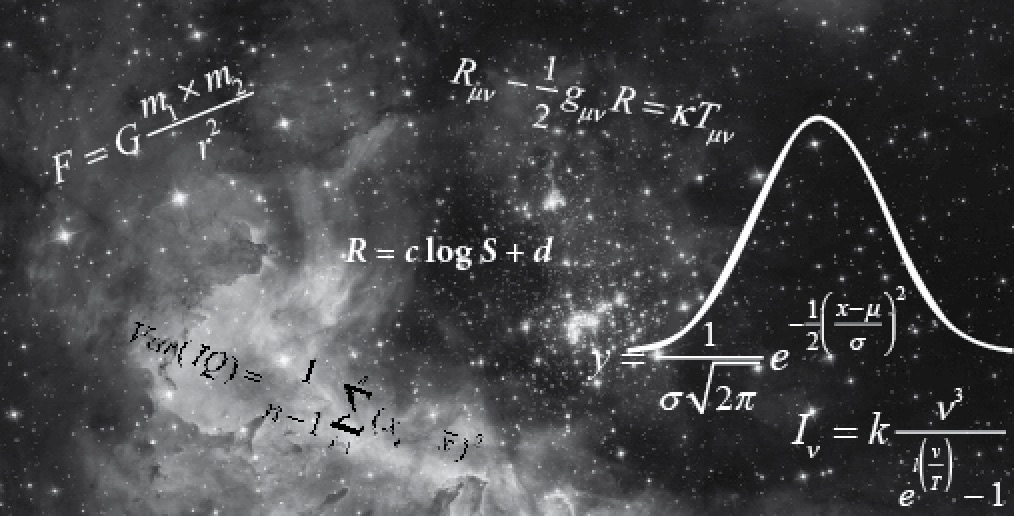
This is an important question that gets to the essence of the differences in intelligence among humans. For this reason, it requires more than a superficial answer, and I ask the reader to bear with me during a longer than usual post, while I share what we know from research. Let’s first look at what makes a concept difficult to comprehend and then examine why people of different IQ’s will experience different levels of difficulty in mastering a particular concept.
What Makes a Concept Difficult to Comprehend?
There are two vital factors that determine the difficulty of a concept:
- The number of subordinate concepts on which an understanding of that concept rests.
2. The level of abstraction required to understand the concept at the deepest level.
A complex concept, like the top row of a brick wall resting on the bricks below it, is supported by the subordinate concepts that underpin it. For example, a deep understanding of Einstein’s General Theory of General Relativity rests upon an understanding of tensor calculus and Riemannian geometry. But both of these bodies of knowledge, are underpinned by many more fundamental concepts such as multi-dimensional spaces and metrics in differential geometry. Consequently, the General Theory of Relativity is difficult to understand at a deep level. That is, concepts that rest on a tall edifice of subordinate concepts correspond to a high brick wall and present a difficult challenge, because they require the mastery of a large number of subordinate concepts.
In addition to the hierarchal dimension of a concept, the difficulty of a concept is also dependent on the level of abstraction that it involves. The reason that most people prefer to read a tabloid rather than a textbook involving advanced mathematics derives from the fact that the tabloid deals with concrete, everyday issues of “who did what to whom?” (What Daniel Kahneman referred to as “fast and frugal system 1 thinking.”) The textbook, on the other hand, involves ponderous abstract thought (“system 2 thinking”). Richard Haier, a psychologist and neuroscientist, using positron emission tomography (PET) scans to measure glucose metabolism in the brains of individuals hypothesized that complex tasks, such as those involving abstract “system 2 thinking” consume more of the brain’s glucose reserve than “system 1 thinking.” As Joseph Heath, author of Enlightenment 2.0 asserts:
The mind simply didn’t evolve to support the sort of linear, explicit processing that is the hallmark of rational thought … thus the way your brain feels after writing an exam is like the way your back feels after a long day spent lifting boxes–Neither was designed for the task that it is being asked to perform.
If, indeed, deep abstract thought puts a heavier demand on our cognitive energy, then the degree of abstraction involved in a concept is a significant determinant of how difficult it is to master.
Why might an understanding of a particular concept be related to IQ?
In 1968, American educator Benjamin Bloom proposed that “anyone can learn anything,” and people differ only in the rate at which they learn. Therefore, all students can study the same curriculum, albeit at different rates. It was proposed that any student who failed to achieve mastery of a particular learning outcome could be given additional instruction and then retested, continuing the cycle until mastery was achieved. This approach, later called “mastery learning” flew under several tag lines such as, “Learning is a matter of attitude, not aptitude,” and “There are no bad students, only bad teachers.” Under the mastery learning philosophy of education, the explanation for failure was transferred from the student’s ability to the method of instruction. This belief or ideology asserted that all concepts are within the grasp of all people, and people differed only in the speed at which they learned.
One of the most comprehensive studies of mathematical giftedness was conducted in the Soviet Union between 1955 and 1966, by a team of researchers headed by psychologist Vadim Krutetskii. Since the Soviet philosophy rejected a genetic component of intelligence, Krutetskii and his team acknowledged that some students are intellectually gifted, but they were careful not to suggest a genetic link. They asserted: (See references at the end of this post)
The difference between capable, average, and incapable pupils, as our research permits us to conclude, comes down to the following. In able pupils, associations can be formed “on the spot”; in this sense they are “born,” if one can so express it, already generalized, with a minimal number of exercises. In average pupils these associations are established and reinforced gradually, as a result of a whole series of exercises. They form isolated, concrete associations, related only to a given problem, “on the spot.” Through single-type exercises these associations are gradually transformed into generalized associations. In incapable pupils, even the isolated, concrete associations are formed with difficulty, their generalizations are still more difficult, and sometimes such generalizations do not occur at all.
In their studies, Krutetskii’s research group presented a comprehensive variety of problems to students in grades 5 through 8 and examined their thinking processes. One such problem is the following:
The figures below, viewed from left to right form a series.
Which of the figures, A, B, C, D, or E (below) represents the next figure in the series to maintain the same pattern?
They found that some students were unable to determine a pattern until it was explained to them. They suffered the same difficulties on similar sequencing problems. Other students were able to discern patterns in simple problems of this type, but required help when the sequences were more sophisticated. Still other students, designated as “gifted” were able to quickly identify answer B as the natural successor in the sequence. In yet other sequence problems, they were able to identify alternative generating patterns that could lead to a different answer. In essence, the research showed that students differed significantly in their ability to abstract from single “concrete” examples to general representations.
Competence in solving such problems could be easily dismissed as a “silly” or irrelevant skill that has no bearing on intelligence. Yet, it is this ability to see related patterns that’s at the heart of connecting concepts and ideas, because it is related to the ability to deal with abstraction. That’s why there is a high correlation between performance on IQ tests and success in professional careers. The ability to identify similar patterns in structure is vital to understanding metaphor in literature, to extracting trends from an examination of history and to making connections between disparate fields in science.
Why are some people better at solving abstract problems than others?
Modern neuroscience, using brain scans during cerebral activity, is currently attempting to discover what structural differences in some brains make them faster and more efficient in problem solving and dealing with abstraction. Based on their PET scans, Richard Haier and his research team hypothesize that brains capable of rapid absorption of concepts have a more efficient neural structure and consume less glucose in problem solving activities than the average brain. However, these findings are somewhat tentative and neural functions are so complex, that we still know very little of the mechanisms that underpin intelligence. What we do know from observations, such as those made by Krutetskii and his team, is that people differ significantly in the speed at which they acquire an understanding of concepts and in their capacity to understand abstract ideas.
The higher the “brick wall” corresponding to a particular concept, and the higher its level of abstraction, the fewer the number of people who will be able to understand it. The greater time and effort required to master each of the subordinate concepts becomes increasingly prohibitive for those at the lower end of the IQ scale. While most people of IQ 100 will be able to understand the difference between causation and correlation if it is explained to them, fewer of them will be able to understand the concept of sampling from a statistical distribution, although it will be within the grasp of some. An even smaller portion of people of IQ 100 will be able to understand the General Theory of Relativity, and fewer still, the implications and proof of Gödel’s Incompleteness Theorems. Bloom’s assertion that anyone can learn anything, given enough time, ignores the fact that sometimes this timeframe exceeds a person’s lifespan.
Fortunately, most of these esoteric concepts are not relevant to normal, everyday life. Furthermore, while people of high IQ have easier access to the more sophisticated concepts than do people of average IQ, there will be many things that a person of average intelligence may know that are unknown to a person of high IQ. For more information, visit: I know a 140 IQ is good, but what does it really do? – Intelligence and IQ
References
Krutetskii’s description of the difference between capable and non-capable students is taken from Krutetskii, Vadim A. 1976. The Psychology of Mathematical Abilities in Schoolchildren. Chicago: Chicago University Press. p. 262.
Haier, Richard J. 2017. The Neuroscience of Intelligence. London: Cambridge University Press.
