As we humans looked around at the remarkable complexity of nature, we asked how such order and harmony could have come into being. With the human lifespan merely a single frame in a video of 10,000,000 frames, we were incapable of knowing how all this change came about. It was natural for us to anthropomorphize and conjecture that there was a great infinite creator with a grand architectural plan that was unfolding. Like Voltaire’s pompous Professor Pangloss, we asserted, ‘It is demonstrable that things cannot be otherwise than as they are; for all being created for an end, all is necessarily for the best end. Observe, that the nose has been formed to bear spectacles—thus we have spectacles. Legs are visibly designed for stockings —and we have stockings.’ In other words, God formed the nose to accommodate glasses, rather than the reverse.” In essence, the God proposition was a top-down model of creation, in contrast to the theory of evolution that proposes a bottom-up unfolding.
When Darwin put forth his idea of natural selection, it explained what survived and what perished, but it did not explain the origin of the infinite variety of species from which nature made the selection. As the study of genetics evolved, we continued to ask, “How do the nucleotides composing the genes ‘know’ how to rearrange themselves into a new formats, many of which turn out to be advantageous to survival? Our human lifespan was too short to provide more than a snapshot of evolutionary change.
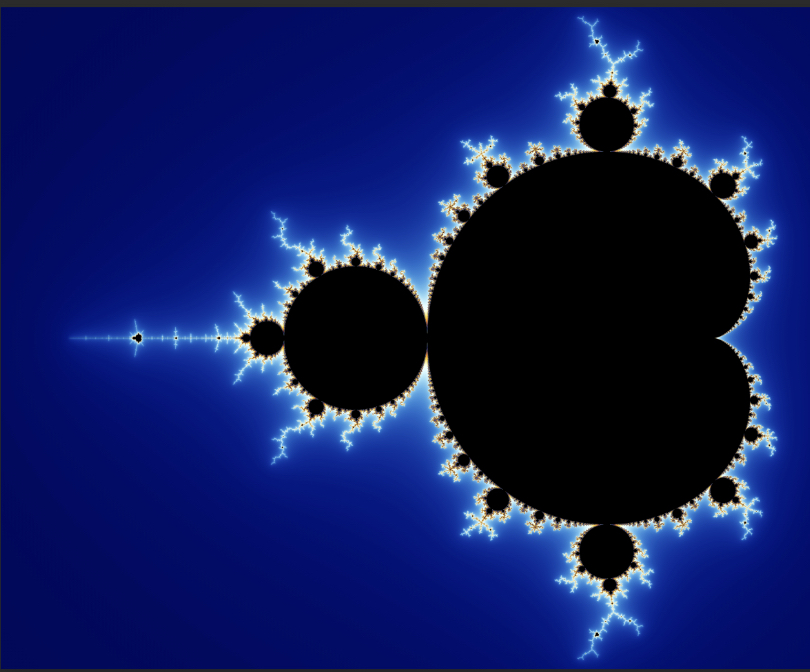
In 1979, maverick mathematician, Benoit Mandelbrot, while working at IBM’s Thomas J. Watson Research Center, took inspiration from an idea of Henri Poincaré, and wrote a computer program to explore the graph that results when the point 0 + 0i in the complex plane is mapped repeatedly under the transformation defined by z → z2 + c where c is a complex number. This involves taking the point 0 + 0i in the complex plane, squaring it, adding c and then iterating this process, ad infinitum, to generate a sequence called the orbit of c. On March 1, 1980, Mandelbrot first glimpsed at the vague outline of what has become known as the Mandelbrot set, shown in the diagram. It is the set of all complex numbers c whose orbit converges i.e., is bounded. This exceedingly complex structure that emerged from that simple iteration ushered in what is now called the mathematics of chaos. When we “zoom in” on this figure, we discover that the boundary of the Mandelbrot set is infinitely “crinkled.” As the value of c changes imperceptibly, the orbit of c changes from a convergent to a divergent series. Mandelbrot and others showed that changing, by a minuscule amount, the initial conditions in a non-linear dynamic system can result in dramatic changes in the outcomes.
By writing a simple program to transform a geometric shape into another shape and then iterating this process many times, Mandelbrot was able to generate so-called Julia sets that resemble forms we see in nature, like trees, broccoli, seahorses or ferns. This similarity between the forms found in nature and Julia sets suggests that nature’s creations, whether they are crystals, clouds, or trees, evolve from a great many iterations of a simple program in a process called “growth.” Since all living things reproduce using DNA, which is a chemical script (or program) for growth, it seemed plausible that computers might be able to simulate life processes.
Before the development of the computer, we humans had no way to visualize what happens when a simple algorithm is replicated a huge number of times. What the Mandelbrot set revealed was that infinite complexity can emerge from simple replications conducted over a long period of time. This enables us to step outside the single frame in our video and simulate how evolution may have unfolded over a 3.7-billion-year period. Of course, none of this addresses where all of the matter and energy in the universe comes from, but that’s a question that is also faced by the God proposition. For more information, visit: Intelligence: Where we Were, Where we Are, & Where we’re Going.
